Figure 1A short story about my favorite audio signal (at the time of writing) for auditioning time smearing in sound systems.

"Transient"

Lasting only for a short time.
- Oxford Languages -

Transient audio signals—rather than sustained signals—are very unforgiving and revealing when auditioning time smearing in, e.g., delay loudspeakers or behind End‑Fire cardioid subwoofer arrays.

Therefore, one might be tempted to think that the most transient, broadband signal, that comes to mind—a perfect (Dirac) impulse that consists of all audible frequencies—might be beneficial.

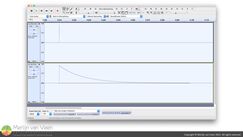
To approximate such a signal in the digital domain, using an audio editor, one simply generates an arbitrary amount of silence, followed by selecting—a single sample—and setting its amplitude to a non‑zero value (Track 1 in Figure 1).

A digital audio signal consisting exclusively of zero‑amplitude samples except for—one sample whose amplitude is non‑zero—is as transient as it gets. Unfortunately, it is also frustratingly silent.

"A white pulse is frustratingly silent."

- Roger Schwenke, Ph.D. -
Honorary MythBuster

The spectrum of such a (im)pulse is white which sounds bright to our non‑linear, logarithmic hearing sense that is less sensitive at low frequencies. From here on, this audio signal will be referred to as "white pulse".

Colors of noise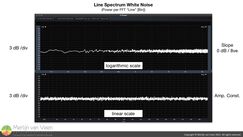

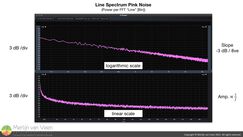Figure 2Figure 3Figure 4Figure 5Figure 6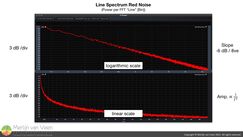Figure 7White noise contains all audible frequencies where each frequency has the same amplitude, giving it a constant power spectral density (Figure 2). As a result, its power per octave band increases at a rate of 3 dB per octave (Figure 3).

After all, there live twice as many frequencies in the octave between 2 kHz and 4 kHz than in the octave between 1 kHz and 2 kHz. Therefore, the former octave contains two times more power than the latter, i.e., 3 dB more power ( 10 × log₁₀ "rule" ).

"White noise draws its name from white light, although light that appears white generally does not have a flat power spectral density over the visible band."

- Wikipedia -

Pink noise, on the other hand, exhibits equal power per octave band (Figure 4) and for that to happen, the amplitudes of its component frequencies need to decrease at a rate inversely proportional to frequency (Figure 5).

There is also red (or Brownian) noise whose power per octave band decreases at a rate of -3 dB per octave (Figure 6) and for that to happen, the amplitudes of its component frequencies need to decrease at a rate inversely proportional to frequency squared (Figure 7).

Unlike white noise that "hisses", red noise "rumbles".

For more information about the colors of noise, read this Wikepedia article.

Red pulse

By passing a white pulse through a first order low‑pass filter—with slope of -6 dB per octave—at a very low corner frequency, the white pulse's power per octave band increase rate of 3 dB is "tilted" into -3 dB per octave band, a red spectrum, hence "red pulse".




As you can tell from the video above (video only, no audio), the red pulse contains more low‑frequency energy, that counterbalances the hearing sense's reduced sensitivity at low frequencies, and—unlike the white pulse—subjectively sounds significantly louder while still remaining very transient due to the leading edge in its waveform (Track 2 in Figure 1).

If there is anything wrong with the timing in your sound system, the red pulse will be most unforgiving and instantaneously reveal any issues—for all audible frequencies—from very low to very high.

When I do not have enough time to fire up the analyzer, this is my go‑to audio signal for finalizing time alignment between loudspeakers by ear. That being said, even when I do have the opportunity to fully calibrate a complete system using an analyzer, I will still "walk the room" afterwards, listening to this signal, while being on the lookout for unexpected surprises.

This audio signal was first brought to my attention by Michal Brůna from Czech Republic during my 2018 seminar there. Since then, it has served me very well. Thank you Michal!

If you wonder what these signals sound like you can download them below. Enjoy, and may they serve you as well as the do me!